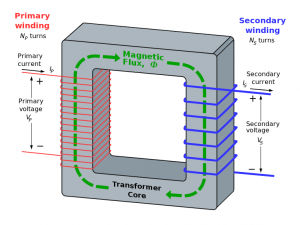
An ideal transformer is a theoretical, linear transformer that is lossless and perfectly coupled; that is, there are no energy losses and flux is completely confined within the magnetic core. Perfect coupling implies infinitely high core magnetic permeability and winding inductance and zero net magneto-motive force.
Ideal transformer equations
By Faraday’s law of induction:

Where Vs is the instantaneous voltage, Ns is the number of turns in the secondary winding, and dΦ/dt is the derivative of the magnetic flux Φ through one turn of the winding. With turns of the winding oriented perpendicularly to the magnetic field lines, the flux is the product of the magnetic flux density and the core area, the magnetic field varying with time according to the excitation of the primary. The expression dΦ/dt, defined as the derivative of magnetic flux Φ with time t, provides a measure of rate of magnetic flux in the core and hence of EMF induced in the respective winding. The negative sign is described by Lenz’s law.

Combining ratio of (1) & (2)

where
- for step-down transformers, a > 1
- for step-up transformers, a < 1
By law of conservation of energy, apparent,real and reactive power are each conserved in the input and output

Combining (3) & (4) with this note (Although ideal transformer’s winding inductance are each infinitely high, the square root of winding inductance’ ratio is equal to the turns ratio.) yields the ideal transformer identity

By Ohm’s law and ideal transformer identity

Apparent load impedance Z‘L (ZL referred to the primary)

Transformer EMF Universal Equation
If the flux in the core is purely sinusoidal, the relationship for either winding between its rms voltage Erms of the winding, and the supply frequency f, number of turns N, core cross-sectional area a in m2 and peak magnetic flux density Bpeak in Wb/m2 or T (tesla) is given by the universal EMF transformer equation:
If the flux does not contain even harmonics the following equation can be used for half-cycle average voltage Eavg of any wave shape:
Source: 1
Discover more from Electrical Engineering 123
Subscribe to get the latest posts sent to your email.



